PESERA-DESMICE: Land management evaluation model

Table of Contents

1. Authors
2. Summary
3. Acronyms
4. Introduction
5. Coarse-scale model description
   1. The PESERA model: summary
   2. Alternative approaches to assessing soil erosion
   3. Process representation in PESERA
   4. Extensions of PESERA
   5. Adapting PESERA
   6. Assessment at catchment scales
6. Cost-effectiveness modelling
   1. Step 1
   2. Step 2
   3. Step 3
   4. Step 4
   5. Step 5
   6. Step 6
   7. Step 7
   8. Step 8
   9. Step 9
   10. Step 10
   11. Step 11
   12. Step 12
7. Agent-Based modelling in Spain
   1. Phase 1
   2. Phase 2
   3. Phase 3
   4. Phase 4
   5. Phase 5
   6. Phase 6
8. Input-Output modelling in Spain
   1. Model construction
   2. Scenario analyses
9. References

Input-Output modelling in Spain

Input-output analysis, initially developed by Wassily Leontief in the late 1930s and still widely used today, is a method to analyse interrelations between sectors of an economy. Inputs and outputs can be of any type, but the most common analyses look at monetary flows between economic sectors and final demand. The basis for input-output analysis is an input-output matrix (see example in Figure 4.4).

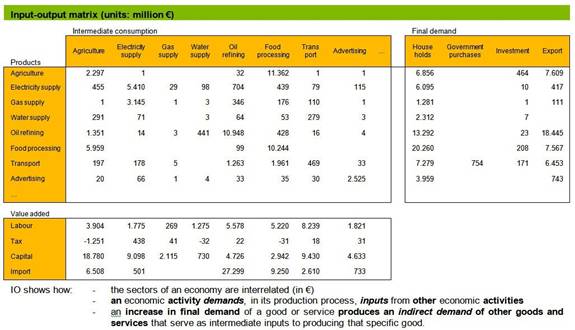
Figure 4.4: An example 8-sector excerpt of an input-output matrix showing inter-industry intermediate consumption and various categories of value added and final demand

To illustrate how input-output analysis works we can take a look at a hypothetical example from one of the economic sectors considered - food processing industries. This would include margarine production plants. To produce a margarine output worth €1.00, the plant would need to source €0.40 of oilseeds from agricultural suppliers (the agricultural sector). It would also need €0.05 worth of electricity, €0.03 worth of gas supply and €0.02 worth of water supply to process the oilseeds into margarine. The factory would have to purchase plastic containers worth €0.10 from the oil refining (incl. plastics) industrial sector. It would also hire the services of a transport sector firm to get oilseeds to the factory and margarine to the distribution channels (€0.05). Finally it would perhaps contract an advertisement firm to set up a publicity campaign to increase output (€0.05). Besides the above intermediate products, the plant would need to pay salaries to its employees (an equivalent €0.07 per €1.00 worth of margarine), pay various taxes (€0.05) and source some inputs not available from the local economy (i.e. the region considered by the input-output analysis) from imports (e.g. palm oil worth €0.15). The shareholders and capital investors in the plant would finally be paid the remaining €0.03.

When one adds all interactions between the various sectors of an economy a matrix results with inter-industry intermediate product (value) fluxes, and value added and final demand categories (Figure 4.4). The example shows how the margarine processing plant would contribute to the column of food processing industries, sourcing various intermediate products and adding value through employment, tax, and interest. In turn, the margarine output of the plant would contribute to the output row of the entire sector, where it is considered as an intermediate product for the economic sectors and different categories of final demand (including household consumption, government purchases, export and investment). Once an entire economy is characterised as a matrix of input-output interrelations, one can use it to perform matrix calculations. If final demand for margarine increases, one can use the matrix to estimate how this will affect the economy. Unitary inputs per output (as in the above example) are called technical coefficients. An increase in margarine production will greatly affect the demand for oilseeds. Producing oilseeds in turn requires increased inputs of machines, fertilisers, etc. After solving the large set of linear equations resulting from a single change (e.g. increased demand for margarine), the impact of that change on the economy can be determined, i.e. the whole supply chain effects are considered potentially including environmental effects.

Originally, natural resources were not taken into consideration in input-output models, but various resources are increasingly accounted for. Guan and Hubacek (2008) review the application of input-output models to water issues, and present a body of research that has developed since the 1980s. Land as a production factor has also been incorporated in input-output models (e.g. Hubacek and Sun, 2001; Hubacek and Giljum, 2003), as have energy use, employment and various types of pollution. While the effect of land degradation on economy has been studied occasionally (e.g. Alfsen et al., 1997 for Ghana; Bandara et al., 2001 for Sri Lanka), inter-sector effects of soil conservation remain, to our knowledge, unstudied to date. The goal of the I/O model described below is to evaluate the wider effects on the regional economy of adopting mitigation strategies for land degradation.

To fill this lacuna, we will develop an input-output model for the Autonomous Region of Murcia, Spain. The model will be coupled to the agent-based model described in section 4.1 and to the modified PESERA model described in section 2 to allow scenario analyses of the regional economic impact of the adoption patterns of remediation technologies, in turn influenced by policy and climate change scenarios.